Current sensor transfer impedance determination method: Difference between revisions
| Line 48: | Line 48: | ||
So 2 ohm: <math>10*^{10}log(\frac{0,08}{50}) \approx -27,96 dB</math> | So 2 ohm: <math>10*^{10}log(\frac{0,08}{50}) \approx -27,96 dB</math> | ||
The difference is <math>-27,96 - (-33,98) = 6,02 dB</math> | The difference to a 1 Ohm impedance is <math>-27,96 - (-33,98) = 6,02 dB</math> | ||
==Conclusion== | ==Conclusion== | ||
Revision as of 16:00, 23 January 2009
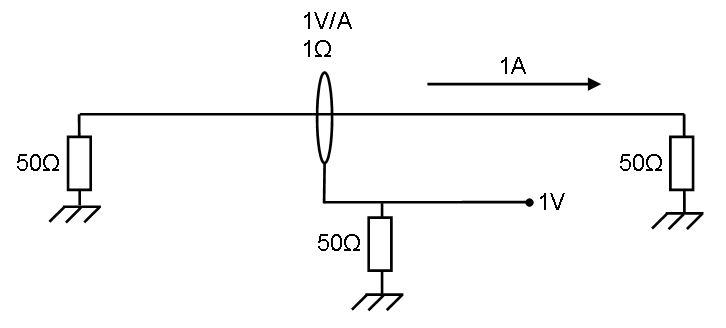
Theory[edit]
The left impedance is the signal generator which is generating enough power for 1 ampere.
This 1Amp. generates in the right impedance.
The current sensor has 1 ohm transfer impedance, this means 1 ampere generates 1 Volt on the measuring part below.
The power in the lower 50 ohm impedance is
So 1 ohm:
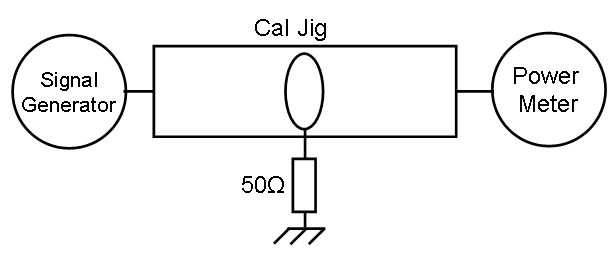
Reference measurement[edit]
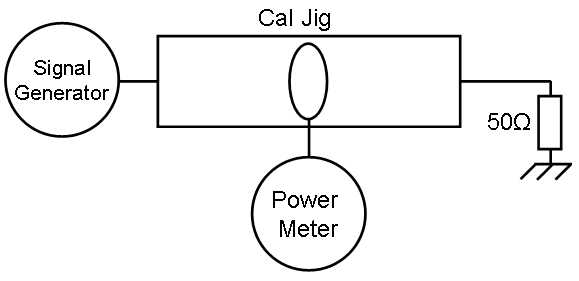
Probe measurement[edit]
Calculation[edit]
and in dBm.
Example[edit]
On 10 MHz we have the following information:
- Calibration: 0 dBm.
- Measurement: -27,96 dBm.
So:
Verification[edit]
The left impedance is the signal generator which is generating enough power for 1 ampere.
This 1 Ampere generates in the right impedance.
The current sensor has 2 ohm transfer impedance, this means 1 ampere generates 2 Volt on the measuring part below.
The power in the lower 50 ohm impedance is
So 2 ohm:
The difference to a 1 Ohm impedance is
Conclusion[edit]
Correction to a 1 ohm impedance is
| Note: | This method is not a replacement for a real calibration as it may be performed by a none traceable device |