Current sensor transfer impedance determination method: Difference between revisions
Jump to navigation
Jump to search
(→Theory) |
|||
| Line 19: | Line 19: | ||
= Calculation = | = Calculation = | ||
<Math>Correction \ factor (dB)= P_{Measured} - P_{Reference} + 33.98</Math> | <Math>Correction \ factor (dB)= P_{Measured} - P_{Reference}+ 33.98</Math> | ||
<Math>P_{Measured}</Math> and <Math>P_{Reference}</Math> in dBm. | <Math>P_{Measured}</Math> and <Math>P_{Reference}</Math> in dBm. | ||
= Example = | |||
On 10 MHz we have the following information: | |||
*Calibration: 0 dBm. | |||
*Measurement: -27,96 dBm. | |||
So: | |||
<math>Imp.=-27,96-0.00+33.98=6,02 dBohm</math> | |||
<math>Imp.= </math> | |||
{{note|This method is not a replacement for a real calibration.}} | {{note|This method is not a replacement for a real calibration.}} | ||
Revision as of 15:45, 23 January 2009
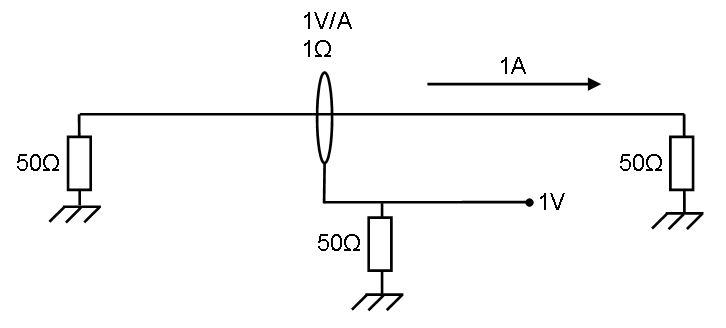
Theory[edit]
The left impedance is the signal generator which is generating enough power for 1 ampere.
This 1Amp. generates in the right impedance.
The current sensor has 1 ohm transfer impedance, this means 1 ampere generates 1 Volt on the measuring part below.
The power in the lower 50 ohm impedance is
So 1 ohm:
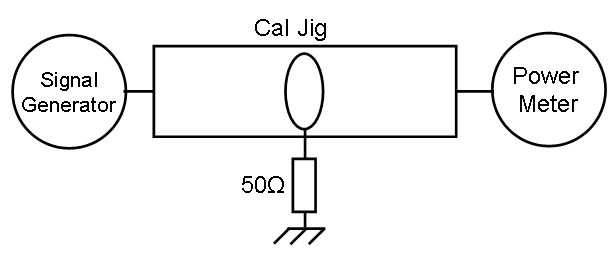
Reference measurement[edit]
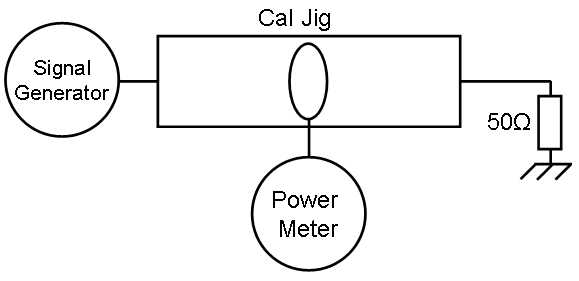
Probe measurement[edit]
Calculation[edit]
and in dBm.
Example[edit]
On 10 MHz we have the following information:
- Calibration: 0 dBm.
- Measurement: -27,96 dBm.
So:
| Note: | This method is not a replacement for a real calibration. |